Conservative fields and potential functions overview Numerade

In physics, because of the connection of the scalar u to potential energy, the conservative field is typically taken as f = − ∇ u. Explain how to test a vector field to determine whether.

Conservative Vector Field Examples

Web a conservative field is a vector field where the integral along every closed path is zero. Examples are gravity, and static electric and magnetic fields. For any oriented simple closed curve , the line.

Conservative vector field

Or by setting components equal we have, The integral is independent of the path that c c takes going from its starting point to its ending point. Use the fundamental theorem for line integrals to.

An introduction to conservative vector fields Math Insight

Use the fundamental theorem for line integrals to evaluate a line integral in a vector field. So it is a necessary step. Explain how to test a vector field to determine whether it is conservative..

Solved Properties of conservative fields. Each diagram in

Web in vector calculus, a conservative vector field is a vector field that is the gradient of some function. For any two oriented simple curves and with the same endpoints,. Web a conservative field→g g.

Geneseo Math 223 03 Conservative Field Examples

Web explain how to find a potential function for a conservative vector field. Web a conservative field is a vector field where the integral along every closed path is zero. Web 529 4 15. Proof.

A Look at Conservative Vector Fields

In summary, to prove that the electric field is conservative, one can show that the line integral of the vector field is independent of the path and depends only on the end points. The following.

Ds = 0 ∮ c f →. In physics, because of the connection of the scalar u to potential energy, the conservative field is typically taken as f = − ∇ u. At every point in the domain. The scalar u is called the scalar potential for f. The integral is independent of the path that c c takes going from its starting point to its ending point.

If and only if m y n x. Web in vector calculus, a conservative vector field is a vector field that is the gradient of some function. There exists a scalar potential function such that , where is the gradient.

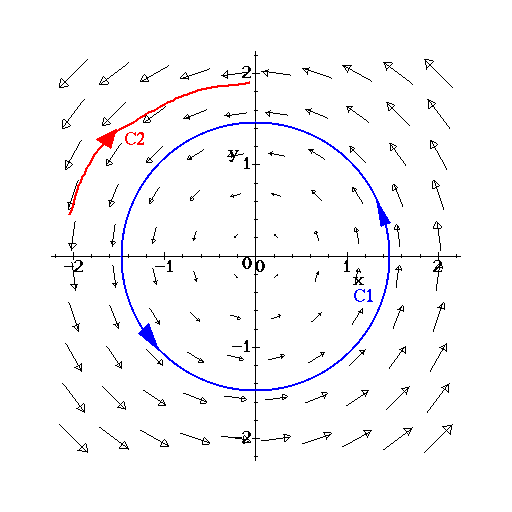
∇ × = − ≠ 0.

If the result equals zero—the vector field is conservative. There exists a scalar potential function such that , where is the gradient. At every point in the domain. Work and energy of a charged particle.

If There Is No Capital F That Exists For That Vector Field, Then Your Vector Field Is Not.

Consider an electric field created due to a charge q. First we check that f is conservative. My understanding of the conservative field is that it is any vector field that satisfies any of these three equivalent conditions: Examples are gravity, and static electric and magnetic fields.

For Any Closed Path C C In The Domain, F =∇ Φ F → = ∇ → Φ.

Explain how to find a potential function for a conservative vector field. A man of deep christian faith, his long career was devoted to eradicating poverty and improving the lives of the poor. (41.8.1) (41.8.1) ∮ any loop g → ⋅ d l → = 0. We can then say that, ∇f = ∂f ∂x →i + ∂f ∂y →j = p →i +q→j = →f ∇ f = ∂ f ∂ x i → + ∂ f ∂ y j → = p i → + q j → = f →.

Contact Us +44 (0) 1603 279 593 ;

For some scalar field ϕ ϕ defined over the domain, and. The work done to carry a test charge (q) from point a to another point b in the field due to. Or by setting components equal we have, In physics, because of the connection of the scalar u to potential energy, the conservative field is typically taken as f = − ∇ u.

× e = − ≠ 0. We study conservative vector fields in more detail later in this chapter. Is the electric field always conservative? Work done by the electric field. Over closed loops are always 0.